Quick and dirty vid on problem solving:
Soon there will be a few more pages devoted to this and more "story problems" on my site.
Right now you can go here for a little bit more: CRHOM Problem Solving.
That page is just the tip of the iceberg. So much to do so little time...
Tuesday, December 22, 2009
Saturday, December 19, 2009
How maths makes the world go round
Always amuses me that people that speak the same language can speak it with subtle differences...like putting an "s" at the end of math and thinking me odd for calling it "the mathematics" instead of just mathematics...anyhow took this from a blog note there is the link to the place I got it, I don't claim to have written it but I do claim fair use. You can take anything off my website or this blog as long as you provide a live link back to the source...
How maths makes the world go round
http://refreshingnews9.blogspot.com/2009/10/how-maths-makes-world-go-round.html
Whether you’re searching for oil, the lost chord or a better kind of carrot, mathematics is the key, says Ian Stewart
Maths makes it: genetic breeding yields a better class of carrot
Like many amateur guitarists, I’d always wondered how to play the opening chord of A Hard Day’s Night. Over the years, I spent hours trying to reconstruct it, but there was something very odd about it: no matter how hard I tried, I could never get it quite right.
In the end, the key to the mystery turned out not to be music, but mathematics. Five years ago fellow Beatles fan and mathematician Jason Brown of Dalhousie University analysed the chord using a method called Fourier analysis, which splits sounds into their basic components. It turns out that the Beatles used a piano as well as their guitars.
It’s not just music that has benefited from a little mathematical knowhow recently. On the sports pages, there has been a bit of fuss about a new type of football, which actually travels in the direction intended. What the reports don’t say is that the design is based on a field of maths called computational fluid dynamics, which uses complicated equations to work out how the air flows past the ball, equations which take into account not just the pattern of the panels, but even details of the seams.
That’s the strange thing about maths. Save for the odd occasion when you want to split the bill at a restaurant, it seems infinitely removed from everyday life. So it comes as a surprise to discover just how much maths is lurking in everyday objects – such as footballs.
We know that maths and technology go hand in hand: the inner workings of Google’s search engine, for example, rely on several areas of advanced maths, such as network theory, matrix algebra and probability theory. The researchers there are highly incentivised to make their work as accurate as possible: improve the maths behind the equations, and oodles more cash floods in from more effective advertising.
But let’s think about something more down to earth: a supermarket vegetable aisle, for example, and in particular, the carrots. The carrot is the second most popular vegetable in the world, after the potato. There are hundreds of varieties, differing in colour, taste, resistance to disease, and ability to survive for weeks in a lorry while being lugged across half of Europe.
All of these types of carrot have been specially bred. One method is to cross-breed different varieties and see what you get; a more modern innovation is genetic engineering. Both rely heavily on maths: it’s used in the statistical calculations required to decide which breed is best, and in the design of the trials that provide the necessary information.
Now, I’d be the first to admit that when you are buying carrots, you don’t need to do that sort of maths. But someone has to, otherwise there wouldn’t be any carrots for us to buy. Old-fashioned breeds don’t work when you have to sell millions of carrots every day. No maths, no veggies.
Anyway, once you’ve lugged that bag of carrots over to the car and dumped it in the boot, you notice that you’re nearly out of petrol. No problem: the supermarket sells that, too. You don’t need to know any maths to stick the nozzle in your car – but without a lot of very difficult maths indeed, there wouldn’t be any petrol in the pump.
Early in September, British Petroleum announced the discovery of a massive new oilfield in the Gulf of Mexico, but you don’t find oil seven miles down by drilling wells at random: you have to know where to look.
Given an accurate map of the rock under the ground, geologists can recognise places where oil may be trapped. But how do you make that map? You make loud bangs at the surface and listen to the returning echoes. By doing the right maths, you can then work out where the different layers of rock are.
It’s a complicated problem, because the echoes from all the different layers of rock interfere with each other. It’s a bit like trying to work out the street plan of a city by shouting loudly and listening to the sounds that bounce off the walls. It has taken decades of work by specialist mathematicians to come up with methods that are practical and accurate: one big oil company now does a quarter of a million of these complex calculations every day.
For centuries, maths has been the main driver of science and technology, and the results have transformed our world. My wife and I have a new grandson, and a few months ago we were able to watch a DVD of him before he was born, made using an ultrasound scan. This employs sound that is so high-pitched that the human ear can’t perceive it. And it works much like oil exploration: the equipment listens to the echoes, and uses maths to reconstruct the shape that must have produced them.
Modern medicine uses many different scanners – CT scans, PET scans, ultrasound. Their common feature is that they use maths to calculate the shape of whatever is being scanned, by analysing the signals that the equipment is designed to detect. The mathematical basis of CT scans was worked out more than a century ago by Johann Radon, a pure mathematician who had no idea that his work – suitably tweaked – would routinely save lives long after he was dead.
Today, medical researchers are developing mathematical ways to detect cancer more accurately. Under a microscope, cancer cells look different from healthy cells, but it takes a trained eye to tell the difference. The mathematics of fractals – very complex geometric shapes – is just what the doctor ordered, helping to capture the difference between the shape of a healthy cell and a cancerous one.
As if that wasn’t enough, maths plays a big part in keeping the environment healthy, too. An example is climate change. Even to detect it, you have to compare what is actually happening with what would have happened if the planet had been left to its own devices. But we can’t rerun the planet’s history, so we have to deduce what would have happened without human intervention. One way we can do that is to model the climate mathematically.
So yes, our fancy electronic gadgets – mobile phones, DVD players, digital cameras, the internet, satnav – rely on a lot of maths. And yes, we use it to make sure that aircraft stay up, Formula 1 cars drive very fast, and gigantic towers don’t collapse. But we seldom realise the extent to which maths has invaded every corner of our lives. It shows up in politics, in opinion polls and focus groups. It controls traffic lights, gets crowds safely into and out of sports stadiums, designs the lenses in our spectacles.
And the reason we don’t notice it is that, entirely sensibly, the maths is kept behind the scenes. If I’m buying carrots, I don’t want to have to learn about the mathematics of genetic trials. If I’m putting petrol in my car, I don’t need to know how to solve the inverse problem for seismic waves. But if I want to understand how my world works, I do need to appreciate that the maths is there. Otherwise, I’ll think that the subject is useless. And if too many of us do that, soon there won’t be enough mathematicians to keep everything working.
Professor Stewart's Hoard of Mathematical Treasures. Published by Profile (RRP £11.99) is available from Telegraph Books at £10.99 + £1.25 p&p. Call 0844 871 1515 or visit books.telegraph.co.uk. He will be speaking at the Royal Society on November 5 at 6.30pm.
How maths makes the world go round
http://refreshingnews9.blogspot.com/2009/10/how-maths-makes-world-go-round.html
Whether you’re searching for oil, the lost chord or a better kind of carrot, mathematics is the key, says Ian Stewart
Maths makes it: genetic breeding yields a better class of carrot
Like many amateur guitarists, I’d always wondered how to play the opening chord of A Hard Day’s Night. Over the years, I spent hours trying to reconstruct it, but there was something very odd about it: no matter how hard I tried, I could never get it quite right.
In the end, the key to the mystery turned out not to be music, but mathematics. Five years ago fellow Beatles fan and mathematician Jason Brown of Dalhousie University analysed the chord using a method called Fourier analysis, which splits sounds into their basic components. It turns out that the Beatles used a piano as well as their guitars.
It’s not just music that has benefited from a little mathematical knowhow recently. On the sports pages, there has been a bit of fuss about a new type of football, which actually travels in the direction intended. What the reports don’t say is that the design is based on a field of maths called computational fluid dynamics, which uses complicated equations to work out how the air flows past the ball, equations which take into account not just the pattern of the panels, but even details of the seams.
That’s the strange thing about maths. Save for the odd occasion when you want to split the bill at a restaurant, it seems infinitely removed from everyday life. So it comes as a surprise to discover just how much maths is lurking in everyday objects – such as footballs.
We know that maths and technology go hand in hand: the inner workings of Google’s search engine, for example, rely on several areas of advanced maths, such as network theory, matrix algebra and probability theory. The researchers there are highly incentivised to make their work as accurate as possible: improve the maths behind the equations, and oodles more cash floods in from more effective advertising.
But let’s think about something more down to earth: a supermarket vegetable aisle, for example, and in particular, the carrots. The carrot is the second most popular vegetable in the world, after the potato. There are hundreds of varieties, differing in colour, taste, resistance to disease, and ability to survive for weeks in a lorry while being lugged across half of Europe.
All of these types of carrot have been specially bred. One method is to cross-breed different varieties and see what you get; a more modern innovation is genetic engineering. Both rely heavily on maths: it’s used in the statistical calculations required to decide which breed is best, and in the design of the trials that provide the necessary information.
Now, I’d be the first to admit that when you are buying carrots, you don’t need to do that sort of maths. But someone has to, otherwise there wouldn’t be any carrots for us to buy. Old-fashioned breeds don’t work when you have to sell millions of carrots every day. No maths, no veggies.
Anyway, once you’ve lugged that bag of carrots over to the car and dumped it in the boot, you notice that you’re nearly out of petrol. No problem: the supermarket sells that, too. You don’t need to know any maths to stick the nozzle in your car – but without a lot of very difficult maths indeed, there wouldn’t be any petrol in the pump.
Early in September, British Petroleum announced the discovery of a massive new oilfield in the Gulf of Mexico, but you don’t find oil seven miles down by drilling wells at random: you have to know where to look.
Given an accurate map of the rock under the ground, geologists can recognise places where oil may be trapped. But how do you make that map? You make loud bangs at the surface and listen to the returning echoes. By doing the right maths, you can then work out where the different layers of rock are.
It’s a complicated problem, because the echoes from all the different layers of rock interfere with each other. It’s a bit like trying to work out the street plan of a city by shouting loudly and listening to the sounds that bounce off the walls. It has taken decades of work by specialist mathematicians to come up with methods that are practical and accurate: one big oil company now does a quarter of a million of these complex calculations every day.
For centuries, maths has been the main driver of science and technology, and the results have transformed our world. My wife and I have a new grandson, and a few months ago we were able to watch a DVD of him before he was born, made using an ultrasound scan. This employs sound that is so high-pitched that the human ear can’t perceive it. And it works much like oil exploration: the equipment listens to the echoes, and uses maths to reconstruct the shape that must have produced them.
Modern medicine uses many different scanners – CT scans, PET scans, ultrasound. Their common feature is that they use maths to calculate the shape of whatever is being scanned, by analysing the signals that the equipment is designed to detect. The mathematical basis of CT scans was worked out more than a century ago by Johann Radon, a pure mathematician who had no idea that his work – suitably tweaked – would routinely save lives long after he was dead.
Today, medical researchers are developing mathematical ways to detect cancer more accurately. Under a microscope, cancer cells look different from healthy cells, but it takes a trained eye to tell the difference. The mathematics of fractals – very complex geometric shapes – is just what the doctor ordered, helping to capture the difference between the shape of a healthy cell and a cancerous one.
As if that wasn’t enough, maths plays a big part in keeping the environment healthy, too. An example is climate change. Even to detect it, you have to compare what is actually happening with what would have happened if the planet had been left to its own devices. But we can’t rerun the planet’s history, so we have to deduce what would have happened without human intervention. One way we can do that is to model the climate mathematically.
So yes, our fancy electronic gadgets – mobile phones, DVD players, digital cameras, the internet, satnav – rely on a lot of maths. And yes, we use it to make sure that aircraft stay up, Formula 1 cars drive very fast, and gigantic towers don’t collapse. But we seldom realise the extent to which maths has invaded every corner of our lives. It shows up in politics, in opinion polls and focus groups. It controls traffic lights, gets crowds safely into and out of sports stadiums, designs the lenses in our spectacles.
And the reason we don’t notice it is that, entirely sensibly, the maths is kept behind the scenes. If I’m buying carrots, I don’t want to have to learn about the mathematics of genetic trials. If I’m putting petrol in my car, I don’t need to know how to solve the inverse problem for seismic waves. But if I want to understand how my world works, I do need to appreciate that the maths is there. Otherwise, I’ll think that the subject is useless. And if too many of us do that, soon there won’t be enough mathematicians to keep everything working.
Professor Stewart's Hoard of Mathematical Treasures. Published by Profile (RRP £11.99) is available from Telegraph Books at £10.99 + £1.25 p&p. Call 0844 871 1515 or visit books.telegraph.co.uk. He will be speaking at the Royal Society on November 5 at 6.30pm.
Thursday, December 17, 2009
Wild About Math
This is a very cool blog, you can end up spending quite a bit of time here if you enjoy math, or things related to it:
WILDAABOUTMATH
I sent him a short email showing why cross multiplication works and he was kind enough to put up a couple links and my vids on his site. I consider it an honor because he has put a lot of effort into his blog and it is a fun place to go. You should check it out when you get the chance. Careful: you can spend a few hours before you know it...
There are lots of math sites and blogs out there. Some are better than others. I have to start going through them along with the websites I frequent and provide links and information on why I like and use them. This should save you time.
Meantime I am looking for even more sites that are good and will start spending the time to contact sites I like and use so we can share links, which I am told is an important part of building free web traffic and getting good ratings with the search engines.
I also am learning about affiliating with various companies to help offer their products and services to people who come to my site...however I am pretty picky because there is so much raw unadulterated CRAP out there for teaching math or "helping" you teach or learn math that is expensive and useless. I am determined to make it so that if you heard about it on this blog or on my site it is not just a good product or service but a FANTASTIC, most extremely excellent product or service. Then the next trick is getting it at a good price. I have decided NOT to rip on the crappy stuff; we'll focus on the positive.
There are a couple rip offs and scams that I will steer you clear of and a couple teaching methods that do about as much harm as good in my experience. I have to do it in such a way as I don't get sued...lol.
Meantime stuff I like is hard to affiliate with...but I'm working on it. Mortensen Math products and Math U See come to mind...
You will start seeing more stuff for sale on my sites and soon there will be a marketplace tab at Crewton Ramone's House Of Math where you can get all manner of math stuff...just fun stuff like math t-shirts or ties and jewelry...as well as books and software and etc. I have even located some cool PDF's for low cost where you can get 90 sheets of math print outs, everything from simple graph paper to fractions and cut out's for math activities...they have previews so you can see what I'm talking about...
Just takes time to build it all. But with winter break coming up I should have more free time to get it done...key word "should." Check out Crewton Ramone on FaceBook...
WILDAABOUTMATH
I sent him a short email showing why cross multiplication works and he was kind enough to put up a couple links and my vids on his site. I consider it an honor because he has put a lot of effort into his blog and it is a fun place to go. You should check it out when you get the chance. Careful: you can spend a few hours before you know it...
There are lots of math sites and blogs out there. Some are better than others. I have to start going through them along with the websites I frequent and provide links and information on why I like and use them. This should save you time.
Meantime I am looking for even more sites that are good and will start spending the time to contact sites I like and use so we can share links, which I am told is an important part of building free web traffic and getting good ratings with the search engines.
I also am learning about affiliating with various companies to help offer their products and services to people who come to my site...however I am pretty picky because there is so much raw unadulterated CRAP out there for teaching math or "helping" you teach or learn math that is expensive and useless. I am determined to make it so that if you heard about it on this blog or on my site it is not just a good product or service but a FANTASTIC, most extremely excellent product or service. Then the next trick is getting it at a good price. I have decided NOT to rip on the crappy stuff; we'll focus on the positive.
There are a couple rip offs and scams that I will steer you clear of and a couple teaching methods that do about as much harm as good in my experience. I have to do it in such a way as I don't get sued...lol.
Meantime stuff I like is hard to affiliate with...but I'm working on it. Mortensen Math products and Math U See come to mind...
You will start seeing more stuff for sale on my sites and soon there will be a marketplace tab at Crewton Ramone's House Of Math where you can get all manner of math stuff...just fun stuff like math t-shirts or ties and jewelry...as well as books and software and etc. I have even located some cool PDF's for low cost where you can get 90 sheets of math print outs, everything from simple graph paper to fractions and cut out's for math activities...they have previews so you can see what I'm talking about...
Just takes time to build it all. But with winter break coming up I should have more free time to get it done...key word "should." Check out Crewton Ramone on FaceBook...
Wednesday, December 16, 2009
"Ten Apples Up On Top..."
Ever since I started this little adventure of blogging and building Crewton Ramone's House of Math I have talked about having links to products I know work (for teaching math) or that I actually use and have had good experiences with.
Here is a super simple one:
For teaching little kids how to count and get a one to one correspondence this book is great. Don't just read it to them, have them count and point with their fingers...get creative. Do simple addition and ask how many more one animal has than the other. Count a lot on just about every page, add up the apples--"How many do they have together?" "Who has the most apples?" etc. There are times when this book takes a half hour to get through...if you just read it it takes less than ten minutes.
Talk about the pictures, count by tens at the end and count by 7's when they all have 7 apples up on top. There is a page where the lion has 3 the dog has 4 and the tiger has 7...make it into a little problem 3 + 4 = 7 but also show them 3 needs 4 to be 7 and 4 needs 3 to be 7 etc. MAIN THING: HAVE FUN.
Add this book to your library if you have young children. As you can see it's a very low cost tool that can make a big difference if used properly. Eventually maybe I'll have a detailed page that shows some of the things I do, page by page, but I'm pretty sure you can handle it. Great before bedtime or as a playtime activity where you get out the blocks and follow along...
EAT SLEEP MATH.
Here is a super simple one:
For teaching little kids how to count and get a one to one correspondence this book is great. Don't just read it to them, have them count and point with their fingers...get creative. Do simple addition and ask how many more one animal has than the other. Count a lot on just about every page, add up the apples--"How many do they have together?" "Who has the most apples?" etc. There are times when this book takes a half hour to get through...if you just read it it takes less than ten minutes.
Talk about the pictures, count by tens at the end and count by 7's when they all have 7 apples up on top. There is a page where the lion has 3 the dog has 4 and the tiger has 7...make it into a little problem 3 + 4 = 7 but also show them 3 needs 4 to be 7 and 4 needs 3 to be 7 etc. MAIN THING: HAVE FUN.
Add this book to your library if you have young children. As you can see it's a very low cost tool that can make a big difference if used properly. Eventually maybe I'll have a detailed page that shows some of the things I do, page by page, but I'm pretty sure you can handle it. Great before bedtime or as a playtime activity where you get out the blocks and follow along...
EAT SLEEP MATH.
Tuesday, December 15, 2009
Cross Multiplication
Introduction:
Building on that short foundation:
Remember, I am condensing hours and hours into less than 20 minutes. If you miss something or you don't "get it" watch them a couple times...
Two vids on Cross Multiplication. More explanation with words and maybe some pictures coming your way soon. Meantime go to Crewton Ramones's House of Math for more.
Also if you think this is cool, wait till you see the vids I make on algebra: problems like (x+1)(x+2) = x2+2x+2, I use exactly the same blocks.
More beginner multiplication at CRHOM.
Building on that short foundation:
Remember, I am condensing hours and hours into less than 20 minutes. If you miss something or you don't "get it" watch them a couple times...
Two vids on Cross Multiplication. More explanation with words and maybe some pictures coming your way soon. Meantime go to Crewton Ramones's House of Math for more.
Also if you think this is cool, wait till you see the vids I make on algebra: problems like (x+1)(x+2) = x2+2x+2, I use exactly the same blocks.
More beginner multiplication at CRHOM.
Monday, December 14, 2009
New Video on Subtraction With Addends
Here is a quick Video explaining the concept of "Small Addition" when doing subtraction.
Go to my website for more.
Go to my website for more.
Friday, December 11, 2009
New Video on Making Change.
If a picture is worth a thousand words...then a video is...
Watch the vid a couple times if it goes too fast. Soon you will be able to make change in your head, fast and easy.
For a bit more go to my subtraction page. My website is slowly but surely growing. Currently I'm in video production mode. Will be adding some videos this week on problem solving, multiplication and cross multiplication...which I keep seeing around the web as a "trick" because they only see the numbers and don't understand what they are counting.
That will take about 3 vids to cover, ten another one on subtraction and some more on algebra...so much to do...
Watch the vid a couple times if it goes too fast. Soon you will be able to make change in your head, fast and easy.
For a bit more go to my subtraction page. My website is slowly but surely growing. Currently I'm in video production mode. Will be adding some videos this week on problem solving, multiplication and cross multiplication...which I keep seeing around the web as a "trick" because they only see the numbers and don't understand what they are counting.
That will take about 3 vids to cover, ten another one on subtraction and some more on algebra...so much to do...
Wednesday, November 25, 2009
The Importance of Addends.
In the course of the last few years, I have taken on students of every skill level and age you can imagine, from 3 to 83, from those who could not yet count to those who just needed some brush up in preparation for calculus. A recurring theme among those students who are getting the poorest grades and saying they aren't "getting it" is the inability to make tens (or nines).
Seems basic enough, but you'd be surprised how many students come to me because they are failing algebra who can't add fractions, multiply quickly and easily in their heads or answer simple questions like 6 plus what makes 10? Seriously, they don't know their addends and their parents are mystified that their smart little 14 year old isn't doing well in algebra, or worse expect they won't do well because they themselves didn't do well, as if poor math skills can "run in the family" due to poor math genes.
The problem especially for girls is once they start getting bad grades in math ALL the grades suffer; not always but certainly often enough and although it is not specific to females, it is more pronounced. When I was traveling the nation I heard that story over and over again from women of all ages: they could point to the year that they got their first failing grade in math as the year that their academic careers either came to an end or as the year that marked beginning of the end. Studies from the University of Women and others have borne this anecdotal evidence out.
There have been long dissertations written as to why this is but the point is that it is a fact not just my opinion and further discussion is beyond the scope of this essay. Let it suffice to say males preserve their ego by thinking there is something wrong with math or the teacher if they don't "get it" while women tend to think "there must be something wrong with me" if they don't get it. This leads to all manner of self esteem issues and to students who graduate high school and then go on to college asking important questions like "what kind of degree can I get out of this university without taking much math?"
Recently I started doing some work at a local intermediate school, grades 6 through 8, where I am exposed to larger groups of students and can notice some trends and commonalities. I set it up so that I was allowed to work with "honors" and "accelerated" students sometimes labeled gifted and talented ("GT" for short) as well as the students who were "challenged" or receiving "F's".
Without exception the F students were unable to easily answer questions like "what does four need to make ten?", or "what does five need to make nine?" Even some of the GT kids were slow to answer or needed the aid of their fingers.
To some this is stunning. I had a parent sit in on their student's first lesson. The parent was mortified to see that when asked some basic addends like those already mentioned and also ones past ten like "what's 6 + 7?" or "8 + 5?" their child either responded incorrectly or took quite a bit of time to arrive at an answer. When presented with a simple fractions problem 1/2 + 1/3 the expression was one of horror. "2/5" was the sheepish reply...
Gee, why are they having trouble in algebra?
I see it all the time. So I simply get back to basics as part of my tutoring. I remind students and parents of the five basic concepts, and make simple addition and subtraction part of the session every time.
The 45 addends are foundational math. It has been said that you can do all math with just addition and subtraction...it just takes longer. Multiplication is just adding repeatedly, division can be thought of as subtracting repeatedly although I personally don't teach it that way. I find that concept based teaching makes learning math much easier but students will still run into trouble if they get bogged down in computation while they are trying to figure out what to do to solve a problem. This applies to all areas of math...whether it's algebra or calculus or just percentages and fractions.
I can teach them what to do, but they still have problems with how to do it because they lack the most basic skill sets. The good news is these skill sets are easily learned and with a little practice easily mastered.
Any student who does not suffer from serious learning disabilities (and even then) can learn to add 3 + 7 to get 10. I have one student who is considered severely disabled but if you ask him any of the combinations for 10 he can tell you without hesitation, and certainly faster than the "normal" students twice his age at the intermediate school.
Knowing all the addends but at leaste the two digit combinations for nine and ten are quite useful for adding any two numbers with ease. Very young students can now see that adding 7 + 5 to get 12 and adding 57 + 5 to get 62 is basically the same problem with some extra tens along for the ride...7 + 5 is always 12; the way I teach them do this is 7 needs 3 to be ten so it takes the 3 out of the 5 and there are 2 left over:
7 + 5 = 7 + (3 + 2) = (7 + 3) + 2 = 10 + 2 = 12 that process takes a long time to type out but takes a fraction of second in your head, once you understand what you are doing.
The next step is subtracting, which the way I teach it is just small addition. I taught a couple of 13 year old girls how to make change for 100.00 They were quite pleased with themselves once they figured out all they had to do was make three nines and a ten. Suddenly a problem like this took seconds:
100.00
-64.57
The way they did it (and this make take you a few seconds to "get") was 6 needs 3 to make 9, 4 needs 5 to make 9, 5 needs 4 to make 9, and 7 needs 3 to make 10. We did it from left to right, not right to left and we didn't count backwards, borrow or otherwise consternate ourselves.
After just a little practice I have students who can tell me the answers to these kinds of problems as fast as I can write them on the board. Often exclaiming "WOW, that's EASY!"
Then we can move on to problems like this:
34
-7
We can't "take 7 out of 4" (subtract 7 from 4) so we take it out of one of the 10s...so skipping a step we just add 3 to 4 and get 27. Instead of counting backwards, we just turned it into a small addition problem...we didn't do 14 minus 7, we did 3 plus 4...ask any kid which is easier.
34
-7
27
Parents and teachers often have a hard time with this one even after they see it a few times, and see more examples. The point is knowing your addends makes subtraction easy and fast once you know how.
It also makes integers easy, negative numbers and their differences are a snap.
I have developed a iphone App that helps students practice the combinations for nines and tens, go to the iapp store and search Crewton Ramone...or go here for CREWTON RAMONE'S ABSOLUTELY AMAZING ADDENDS. Just give me your email address and I'll send you a link where you can play with it online, or download it to your Mac or PC for FREE.
In another article I will go on to show how knowing your addends makes learning multiplication much easier too. Addends along with simple patterning make multiplication simple, and multiplication is the first "milestone" in the mathematics because it allows you to count very, very quickly.

Seems basic enough, but you'd be surprised how many students come to me because they are failing algebra who can't add fractions, multiply quickly and easily in their heads or answer simple questions like 6 plus what makes 10? Seriously, they don't know their addends and their parents are mystified that their smart little 14 year old isn't doing well in algebra, or worse expect they won't do well because they themselves didn't do well, as if poor math skills can "run in the family" due to poor math genes.
The problem especially for girls is once they start getting bad grades in math ALL the grades suffer; not always but certainly often enough and although it is not specific to females, it is more pronounced. When I was traveling the nation I heard that story over and over again from women of all ages: they could point to the year that they got their first failing grade in math as the year that their academic careers either came to an end or as the year that marked beginning of the end. Studies from the University of Women and others have borne this anecdotal evidence out.
There have been long dissertations written as to why this is but the point is that it is a fact not just my opinion and further discussion is beyond the scope of this essay. Let it suffice to say males preserve their ego by thinking there is something wrong with math or the teacher if they don't "get it" while women tend to think "there must be something wrong with me" if they don't get it. This leads to all manner of self esteem issues and to students who graduate high school and then go on to college asking important questions like "what kind of degree can I get out of this university without taking much math?"
Recently I started doing some work at a local intermediate school, grades 6 through 8, where I am exposed to larger groups of students and can notice some trends and commonalities. I set it up so that I was allowed to work with "honors" and "accelerated" students sometimes labeled gifted and talented ("GT" for short) as well as the students who were "challenged" or receiving "F's".
Without exception the F students were unable to easily answer questions like "what does four need to make ten?", or "what does five need to make nine?" Even some of the GT kids were slow to answer or needed the aid of their fingers.
To some this is stunning. I had a parent sit in on their student's first lesson. The parent was mortified to see that when asked some basic addends like those already mentioned and also ones past ten like "what's 6 + 7?" or "8 + 5?" their child either responded incorrectly or took quite a bit of time to arrive at an answer. When presented with a simple fractions problem 1/2 + 1/3 the expression was one of horror. "2/5" was the sheepish reply...
Gee, why are they having trouble in algebra?
I see it all the time. So I simply get back to basics as part of my tutoring. I remind students and parents of the five basic concepts, and make simple addition and subtraction part of the session every time.
The 45 addends are foundational math. It has been said that you can do all math with just addition and subtraction...it just takes longer. Multiplication is just adding repeatedly, division can be thought of as subtracting repeatedly although I personally don't teach it that way. I find that concept based teaching makes learning math much easier but students will still run into trouble if they get bogged down in computation while they are trying to figure out what to do to solve a problem. This applies to all areas of math...whether it's algebra or calculus or just percentages and fractions.
I can teach them what to do, but they still have problems with how to do it because they lack the most basic skill sets. The good news is these skill sets are easily learned and with a little practice easily mastered.
Any student who does not suffer from serious learning disabilities (and even then) can learn to add 3 + 7 to get 10. I have one student who is considered severely disabled but if you ask him any of the combinations for 10 he can tell you without hesitation, and certainly faster than the "normal" students twice his age at the intermediate school.
Knowing all the addends but at leaste the two digit combinations for nine and ten are quite useful for adding any two numbers with ease. Very young students can now see that adding 7 + 5 to get 12 and adding 57 + 5 to get 62 is basically the same problem with some extra tens along for the ride...7 + 5 is always 12; the way I teach them do this is 7 needs 3 to be ten so it takes the 3 out of the 5 and there are 2 left over:
7 + 5 = 7 + (3 + 2) = (7 + 3) + 2 = 10 + 2 = 12 that process takes a long time to type out but takes a fraction of second in your head, once you understand what you are doing.
The next step is subtracting, which the way I teach it is just small addition. I taught a couple of 13 year old girls how to make change for 100.00 They were quite pleased with themselves once they figured out all they had to do was make three nines and a ten. Suddenly a problem like this took seconds:
100.00
-64.57
The way they did it (and this make take you a few seconds to "get") was 6 needs 3 to make 9, 4 needs 5 to make 9, 5 needs 4 to make 9, and 7 needs 3 to make 10. We did it from left to right, not right to left and we didn't count backwards, borrow or otherwise consternate ourselves.
100.00 -64.57 35.43
After just a little practice I have students who can tell me the answers to these kinds of problems as fast as I can write them on the board. Often exclaiming "WOW, that's EASY!"
Then we can move on to problems like this:
34
-7
We can't "take 7 out of 4" (subtract 7 from 4) so we take it out of one of the 10s...so skipping a step we just add 3 to 4 and get 27. Instead of counting backwards, we just turned it into a small addition problem...we didn't do 14 minus 7, we did 3 plus 4...ask any kid which is easier.
34
-7
27
Parents and teachers often have a hard time with this one even after they see it a few times, and see more examples. The point is knowing your addends makes subtraction easy and fast once you know how.
It also makes integers easy, negative numbers and their differences are a snap.
I have developed a iphone App that helps students practice the combinations for nines and tens, go to the iapp store and search Crewton Ramone...or go here for CREWTON RAMONE'S ABSOLUTELY AMAZING ADDENDS. Just give me your email address and I'll send you a link where you can play with it online, or download it to your Mac or PC for FREE.
In another article I will go on to show how knowing your addends makes learning multiplication much easier too. Addends along with simple patterning make multiplication simple, and multiplication is the first "milestone" in the mathematics because it allows you to count very, very quickly.

Thursday, November 12, 2009
Days fly by...
I have started teaching kids at a public school. 1/2 before school and 1/2 hour after on Tue, Wed and Thur. So far it's been fun but the time constraints make it a little difficult.
More on this and what I do for an half hour shortly.
More on this and what I do for an half hour shortly.
Tuesday, October 27, 2009
Time Flies When Your Having Math
2 weeks since my last blog post. What a lot of math has transpired in that time. I have managed to add some students and lose some students. Have started doing some work with a local school here doing math before and after school in short half hour segments.
Teaching math today, all day. In my first class a seven year old had a secret he wanted to tell me. I said tell me your secret later lets do math. All through the class he kept telling me he wasn't going to tell me his secret...I kept telling him it was fine I didn't want to know his secret, I wanted to do math. (counting by threes at this point).
At last he couldn't stand it anymore. "I'm gonna tell you my secret."
"Ok"
"You are never alone," he half whispers. "The earth talks to you. The earth talks to you, so you are never alone."
"Really?"... Read More
"Really. Does that make you happy?"
"Yes."
"Good: it should."
Back to math...
Another class with the younger students...the child in this picture is 4. He can tell you the quadratic formula if you ask him, and he can tell you how many 4's in 32.

All we are doing is counting and comparing. He can see quite easily that there are eight 4s in 32. He doesn't even have to count by fours although he can thanks to multiplication rock and the Mortensen Skip Count songs...before we did 32, we did 12, and 20. The word "division" didn't even come up...we can introduce that concept later.
The three year old who has motor skills that are not as refined as his brother's had his own blocks to measure...he was counting how many 3's were in 12 at the same time as his brother was measuring 32. They had been working together but then they each got their own "problem" to solve. He was happy to report that there really are four threes in 12. And that four 3s is the same as 12.

Note how I have the blocks pushed up against the tray so they can't get away from him as he lines them up: put the child in a situation where they can not fail.
Now they can take the blocks and build towers with them...this requires motor skills and as they build I ask how many blocks they have AND how many they have. In other words we count three blocks and that's the same as 12 since he is using 4s.

The towers fall over several times but it's no big deal, we just build it and count it again. In this case we are only using the blocks we measured with...

So the three year old only has to stack a few blocks, he measured 15 next and then built his tower. He only had five blocks to deal with. The look of satisfaction on his face when he got then to stay after he let go of them was priceless.
All at once we develop self esteem, fine motor skills, counting, and multiplication and set up for future division concepts all in one easy lesson.
Teaching math today, all day. In my first class a seven year old had a secret he wanted to tell me. I said tell me your secret later lets do math. All through the class he kept telling me he wasn't going to tell me his secret...I kept telling him it was fine I didn't want to know his secret, I wanted to do math. (counting by threes at this point).
At last he couldn't stand it anymore. "I'm gonna tell you my secret."
"Ok"
"You are never alone," he half whispers. "The earth talks to you. The earth talks to you, so you are never alone."
"Really?"... Read More
"Really. Does that make you happy?"
"Yes."
"Good: it should."
Back to math...
Another class with the younger students...the child in this picture is 4. He can tell you the quadratic formula if you ask him, and he can tell you how many 4's in 32.

All we are doing is counting and comparing. He can see quite easily that there are eight 4s in 32. He doesn't even have to count by fours although he can thanks to multiplication rock and the Mortensen Skip Count songs...before we did 32, we did 12, and 20. The word "division" didn't even come up...we can introduce that concept later.
The three year old who has motor skills that are not as refined as his brother's had his own blocks to measure...he was counting how many 3's were in 12 at the same time as his brother was measuring 32. They had been working together but then they each got their own "problem" to solve. He was happy to report that there really are four threes in 12. And that four 3s is the same as 12.

Note how I have the blocks pushed up against the tray so they can't get away from him as he lines them up: put the child in a situation where they can not fail.
Now they can take the blocks and build towers with them...this requires motor skills and as they build I ask how many blocks they have AND how many they have. In other words we count three blocks and that's the same as 12 since he is using 4s.

The towers fall over several times but it's no big deal, we just build it and count it again. In this case we are only using the blocks we measured with...

So the three year old only has to stack a few blocks, he measured 15 next and then built his tower. He only had five blocks to deal with. The look of satisfaction on his face when he got then to stay after he let go of them was priceless.
All at once we develop self esteem, fine motor skills, counting, and multiplication and set up for future division concepts all in one easy lesson.
Tuesday, October 13, 2009
Dragon Tales or Math?
One child three the other four. Watching the same PBS' Dragon Tales episode for the tenth time...
Off goes the teevee. The children wail. "I don't wanna play math...!!!"
"I don't wanna watch this show again and this is a benign dictatorship not a democracy."
I'd have pictures but they were butt naked and I have been getting a ration for posting pics of naked math students even though they are under 5...sick world we live in, but I digress.
I got out a ten and a two. Laid them lengthwise and said measure that with fours...the older boy gets out the blocks and carefully lays them along side.
Laughs when he lines them up and counts them..."four!" He says triumphantly. But then he sees I am not approving.
"Count it again..."
Counts carefully.
"...three I mean three!"
Self correcting, no "no" in the lesson. We are about to put away the ten and two but the three year old exclaims "I want to count it!!!"
He counts the fours and agrees there are three there. Three 4s is the same as a ten and a two. Next, I got out a two tens and a four...measure with sixes. They got out sixes and lined them up next to the tens and four...
This takes some motor skills and the 3 year old was happy to let his brother line them up nicely while he got out the blocks. They counted. "Four!!!"
"Very good. Four 6s is exactly the same as two tens and four."
"And two sixs is 12!!!" He says after comparing the two sixes to the two tens and seeing that he used up a ten and two of the units on the next ten.
Okay lets build tens...they each got their on ten section of the top tray and I made the older one build his own but set up the blocks for the younger one where all he had to do was match the right block to complete the ten.
Then we got on the computer and built nines and tens...they had fun and I didn't have to watch re-runs. I was using the Freeware version, so it had nines and tens, there are links on this page to a demo that only builds tens too...the software is about to get a slight upgrade where every time you get it right you get some audio reinforcement too. For now YOU can provide it for them, and never say no that's not right, if they get it wrong they are just getting more information so you can say, "try again" or "too much", or needs to be bigger or what have you.
Tweet
Off goes the teevee. The children wail. "I don't wanna play math...!!!"
"I don't wanna watch this show again and this is a benign dictatorship not a democracy."
I'd have pictures but they were butt naked and I have been getting a ration for posting pics of naked math students even though they are under 5...sick world we live in, but I digress.
I got out a ten and a two. Laid them lengthwise and said measure that with fours...the older boy gets out the blocks and carefully lays them along side.
Laughs when he lines them up and counts them..."four!" He says triumphantly. But then he sees I am not approving.
"Count it again..."
Counts carefully.
"...three I mean three!"
Self correcting, no "no" in the lesson. We are about to put away the ten and two but the three year old exclaims "I want to count it!!!"
He counts the fours and agrees there are three there. Three 4s is the same as a ten and a two. Next, I got out a two tens and a four...measure with sixes. They got out sixes and lined them up next to the tens and four...
This takes some motor skills and the 3 year old was happy to let his brother line them up nicely while he got out the blocks. They counted. "Four!!!"
"Very good. Four 6s is exactly the same as two tens and four."
"And two sixs is 12!!!" He says after comparing the two sixes to the two tens and seeing that he used up a ten and two of the units on the next ten.
Okay lets build tens...they each got their on ten section of the top tray and I made the older one build his own but set up the blocks for the younger one where all he had to do was match the right block to complete the ten.
Then we got on the computer and built nines and tens...they had fun and I didn't have to watch re-runs. I was using the Freeware version, so it had nines and tens, there are links on this page to a demo that only builds tens too...the software is about to get a slight upgrade where every time you get it right you get some audio reinforcement too. For now YOU can provide it for them, and never say no that's not right, if they get it wrong they are just getting more information so you can say, "try again" or "too much", or needs to be bigger or what have you.
Tweet
Thursday, October 8, 2009
Continuing Down The Algebra Path

Again this work was done by a 7 year old. The first two are familiar to him he did them with ease and the third one was new ad he had to do it all be himself. Zero help from me.
Then we went into a lesson about concept number two: the highest number we count to is NINE, we only count SAME kind, and the numbers just tell you how many; the places tell you what kind, in algebra the symbols (x, y, x2 etc.) tell you what kind the numbers tell you how many and again we can only count same kind. This also applies to fractions. Understanding the five concepts and making sure they understand how they relate to each part of the math is crucial.

Now that we understand where we are and where we are going and why, a set of problems arranged by degree of difficulty is given. The point of this lesson is to go from drawing to symbols so that they can eventually go from symbols to drawing with ease. We want them to go back and forth easily. They have the ability to do this because the only skill they really need is the ability to count. Then we can re-inforce addends, simple multiplication, factoring and lastly factoring trinomials.

Right now this child is getting comfortable with trinomials and when we move on to explain the distributive theory of multiplication as it applies to two binomials, it's going to be simple and OBVIOUS. Right now we are "just counting".
Earlier in the lesson we built all the addends to 18, and practiced the patterns for multiplication for 2, 3, 4 and 5 by counting as we built towers using the same blocks.

Again the time flew by and best of all we had FUN.
More Algebra on Crewton Ramone's House of Math website.
Just donate a buck thirty three...
Tutoring in the Language of Math
Math is fun. Or it can be if you do it right. Here is a 10 year old who orginally thought math "Sucked." Now he's trying to get me to get his Grandma to pay for lessons twice a week instead of just once a week. Imagine that.
In this lesson we fooled around with subtraction, addends, and algebra. All in one lesson, with simple easy transitions to each.
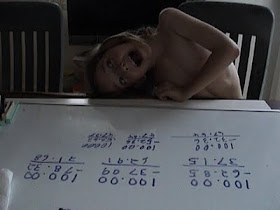
Here we were making change for 100.00 using his new found knowledge of addends suddenly doing these problems was EASY and a snap. Note the notation...no crossing out nothing confusing just clean crisp answers...how do you do it? Make a 9, make a 9, make a 9, make a 10. SIMPLE
Go to my website for a lesson on subtraction. The page is not anywhere near finnished yet but it will give you more..find your way to my Myspace blog too.

Here we go directly into algebra, but you can see we were building the addends past ten, and that oddly enough since math is a language it all goes together and makes SENSE. The algebra he was doing was to cement the work he just did with addends AND to teach factoring...because factoring trinomials is just the clever use of addends and multiplication. An hour went by INSTANTLY. He was quite literally shocked when he realized it was time to go...
Follow the advice of P.T. Barnum "Always leave them wanting more..."
In this lesson we fooled around with subtraction, addends, and algebra. All in one lesson, with simple easy transitions to each.

Here we were making change for 100.00 using his new found knowledge of addends suddenly doing these problems was EASY and a snap. Note the notation...no crossing out nothing confusing just clean crisp answers...how do you do it? Make a 9, make a 9, make a 9, make a 10. SIMPLE
Go to my website for a lesson on subtraction. The page is not anywhere near finnished yet but it will give you more..find your way to my Myspace blog too.

Here we go directly into algebra, but you can see we were building the addends past ten, and that oddly enough since math is a language it all goes together and makes SENSE. The algebra he was doing was to cement the work he just did with addends AND to teach factoring...because factoring trinomials is just the clever use of addends and multiplication. An hour went by INSTANTLY. He was quite literally shocked when he realized it was time to go...
Follow the advice of P.T. Barnum "Always leave them wanting more..."
Friday, October 2, 2009
Simple Multip[lication
Little boys up early, they can watch TV or you can go to my site and watch Multiplication Rock and then watch TV...the 3 year old can sing along to several songs now...beats the heck out of a lot of the stuff that's out there.
Multiplication just allows us to count fast. This will be useful later so we don't get lost in computation when we are doing story problems or so called "higher math" and such.
The lesson lasted about 18 min...6 songs. Got out blocks as we sang...for "I got six" got out sixes...showed 3 sixes is a ten and an eight right along to the song...handed the three year old fours as we sang about 4's...
It's not rocket science. Repetition...breeds speed and skill. They listen to Crazy Frog a hundred times a week why not help them listen to math...instead of or in addition to...?
Multiplication just allows us to count fast. This will be useful later so we don't get lost in computation when we are doing story problems or so called "higher math" and such.
The lesson lasted about 18 min...6 songs. Got out blocks as we sang...for "I got six" got out sixes...showed 3 sixes is a ten and an eight right along to the song...handed the three year old fours as we sang about 4's...
It's not rocket science. Repetition...breeds speed and skill. They listen to Crazy Frog a hundred times a week why not help them listen to math...instead of or in addition to...?
Tuesday, September 29, 2009
Math Lesson 29 Sept 2009

This is the house that math built. Looks like a pile of blocks but it was quite a feat of engineering...lots and lots of math went into it on the subconscious level but also on the very conscious level, as he figured out how to make the roof, how many he needed to make it even on both sides etc. A few questions from me also helped make this a learning experience as well as a reward for doing lots of math problems. NOTE the square root problems under it...we of course counted the sides but before this it was a simple lesson on multiplication and counting by 2s and 5s...

This shows how we don't stop at 9 nor even 12...we have multiplication to 16 here...and these squares were counted in many ways. First we counted to 32 by 2s, then by 8's...and to 48 by 3s and then by 12's. He found counting by 12 was actually pretty easy because "it's just like counting by ones and twos...12, 24, 36, 48!"
Then we counted square roots. The symbols are sloppy but readable and again the child is but SEVEN...! Writing out that the square root of 32 is 4 square roots of 2 was easy and redundant...same with the square root of 48...4 square roots of 3. "This math stuff is easy."
Before all this we built a full top tray of addends, then did some 100.00 minus xx.xx and then some two digit subtraction...we did a whole lot of problems in a short period of time and the reward was the house...Crewton Ramone's House of Math.
Wednesday, September 23, 2009
6hrs 9 students....

A new student looks at Algebra in a new way for the first time. "Here is the expression build me a rectangle." However long it takes them to discover how to do it is how long I wait. Over the years I have learned that I can tell one hell of a lot about a student (or a person) by the way they do this. It is crucially important that they do this for themselves; directed discovery, not memorization and formulae.
Ran the full gamut yesterday, from Counting thru Calculus. Made me come up with a few lessons for little kids to prepare them for Calc and even teach them some basic calc concepts...like distance formula and derivatives via building squares and Pythagoras. Always comes back to the five concepts...and their application. ALWAYS.
Also have an article about critical thinking skills and their development with regard the mathematics banging around in my head.
Am I ever gonna finish a post? Hopefully starting them will cause me to get around to finishing them...need links and more pics...
Wednesday, September 16, 2009
Playing Math For Fun And Profit

Since that square root video I made in the last post many classes have taken place. In this post, I will focus on the classes that used algebra for the primary cross concept method. I use it to teach addition, multiplication, division, factoring and "algebra." Occasionally I use it to teach other concepts as well, but today we focus on basic operations.

The work above was done by a seven year old male student. Our focus was on addition and then on multiplication and lastly on algebra.
The idea is simple. Algebra is abstract mathematics. This method makes the abstract concrete, therefore we can start teaching concepts using algebra. We start simple and work our way up to the complex, keeping the 5 basic concepts in mind and using three period lessons as needed to make sure everyone is on the same page as it were. This is CONCEPT based teaching NOT memorization or formula based teaching. I teach by example with the understanding that the first three times are the baby steps. First time is new. Second time is familiar. Third time is "we did this already, let me do it..."

These two "simple" factoring problems are easy to solve once they have te blocks to work with. The point is not to amaze and astonish the stodgy, well entrenched teachers of mathematics by training monkeys to factor polynomials. The point is to make impressions on the child's memory. It takes about 18 impressions to get a fact from the short term to the long term memory. Some methods use wrote drills, where the child is subjected to seemingly endless worksheets. Here, when they factor
x2 + 7x + 12
They learn that 4 + 3 = 7 and that 4 x 3 = 12 simple enough. They also learn that if the whole rectangle is 12 and one side is 3 the other side must be 4...lastly they can see that if the whole thing is x2 + 7x + 12 the factors are (x + 3)(x +4), which means that if the whole thing is x2 + 7x + 12 and one side is (x + 3) the other side must be (x + 4).
There is a whole lot of math going on in one problem...and they learn and discover all by themselves. Further the trial and error teaches them quite a bit also, and in this method they are not wrong just acquiring more information, because the model is self-correcting due to the fact that success means a rectangle is formed. Hence the slogan "if you can count to nine, tell if something is same or different or not and identify a rectangle, we can teach you math."

Here we have some team work going on. 4 students, 2 teams. The students are racing to try and finish this battery of problems:
x2 + 7x + 12 x2 + 9x + 18
x2 + 9x + 14 x2 + 10x + 25
x2 + 10x + 30 x2 + 12x + 32
They have to factor them and draw a picture in their notebooks. There is an age range of 8 to 14 in this group. Each students is getting a little something different from this exercise, the youngest are still working on addition and multiplication facts, the older students are "doing algebra" all of them are building self esteem and confidence because they "know" algebra is supposed to be hard.
These problems got done a lot faster because they were racing, which is why I like a group as compared to solo lessons.
This post is FAR from finished...
Tuesday, September 8, 2009
Square Numbers
This is a quick video I did on square numbers.
This was a "one take Eddie" but I think it was good enough to get the point across that when we talk about square numbers and expressing the terms as a radical we are simply counting the sides of squares.
This is the beginning of Crewton Ramone's Supremely Simple Square Roots. I plan on making a simple concise Manual with a DVD available that covers square roots, how to square numbers and etc. It will contain a few "tricks" but mostly show the concepts. It will also contain the cross teaching techniques for multiplication...
This was a "one take Eddie" but I think it was good enough to get the point across that when we talk about square numbers and expressing the terms as a radical we are simply counting the sides of squares.
This is the beginning of Crewton Ramone's Supremely Simple Square Roots. I plan on making a simple concise Manual with a DVD available that covers square roots, how to square numbers and etc. It will contain a few "tricks" but mostly show the concepts. It will also contain the cross teaching techniques for multiplication...
Wednesday, September 2, 2009
Algebra is child's play.
So much to do so little time. I just wanted to put this down before it slips away. I did a class with a 7 year old boy. When I met him he couldn't count to 20.
I put
x2+5x+6
on a white board. He didn't get out blocks, he didn't draw pictures he just looked at it for a moment and said "that's gonna be x+2 across and x+3 up...."
And he looked at me funny when he saw that I was a little choked up...now these are the kind of tears that should be associated with learning algebra not the kind that you get in public schools where kids literally jump out of buildings because they hate their math...
I put
x2+5x+6
on a white board. He didn't get out blocks, he didn't draw pictures he just looked at it for a moment and said "that's gonna be x+2 across and x+3 up...."
And he looked at me funny when he saw that I was a little choked up...now these are the kind of tears that should be associated with learning algebra not the kind that you get in public schools where kids literally jump out of buildings because they hate their math...
Monday, August 31, 2009
Algebra teaches addition and multiplication facts.
I now have a seven year old doing algebra...without fear or tears. At the end of this post you'll find a link to a page where children as young as 4 and 5 are taught math with algebra.

We started with an easy one... x + 4x = ___
This often throws a lot of kids off.
x + 4x = 5x
Here it's obvious. The questions that come up for "regular" algebra teachers rarely come up at all because it's visually obvious that "x" means "one x" and you don't need to write 1x because it's redundant. This quick lesson saves a lot of pain later. Now what if each x is 2? Or 5? Easy. X can be anything.

By now, it's getting to the "no big deal" stage. Take a look at the problems below; he did them one by one...and approached them with supreme confidence that he could do it. In fact, it was basically a game of solving the puzzles I made.

We certainly didn't start here; but hour after hour--little by little--each concept compounds and we are now at the point where I just write the symbols and he does the rest. Note: I am teaching him much more than factoring. He is still learning addition facts and multiplication facts AT THE SAME TIME he is learning to factor polynomials. He took more than half an hour to do these four problems, and he had to do a lot of math to solve them.
Expect lots of trial and error; he learned several ways that didn't work for these problems but gained information that will come in handy later.
Take the third expression for example:
x2 + 9x + 20.
He discovered several ways to break up 9, before he got to 4 and 5.
4 + 5 = 9 and 4 x 5 = 20,
we also discussed the economy counting disguised as "fewest pieces possible" so we used four 5's instead of five 4's which is still a little amazing to a 7 year old. He figured out right away that two 10's wouldn't work.
At the end he was much more impressed that he could make 8's by drawing figure 8"s instead of by drawing two circles...


...than the fact that he had factored x2 + 11x + 28! I know 17 year olds who run away in fear when confronted with these kinds of problems, and college graduates who can't remember how to do this...but they paid for the credits anyway.
He also drew a picture of each problem. Drawing is crucial for understanding why we use symbols. It brings the point home that the symbols are faster and that the mathematics expresses reality numerically. He won't soon forget because he knows what the symbols mean and what they look like. The hardest part was counting out 28...what to use? 9's? 8's?...ah hah! 7's.
In the process he figured out some multiplication facts for 8's and 9's...on his way to discovering he needed four 7's...he also made comments like, "this number isn't square...if we had 12x we could make a square number..."
Here is a Polynomial PDF to practice all 45 addends and many multiplication facts too.
Most importantly, we had fun the whole time. Since he stayed on task the whole time and since we did a lot of math very quickly, he got some free time to draw. He was much more impressed with his ability to make 8's and 2's than his ability to factor the trinomial! And the pirate ship came out pretty cool too.


Here is a post with lots of video showing ALGEBRA with toddlers.
Tweet

We started with an easy one... x + 4x = ___
This often throws a lot of kids off.
x + 4x = 5x
Here it's obvious. The questions that come up for "regular" algebra teachers rarely come up at all because it's visually obvious that "x" means "one x" and you don't need to write 1x because it's redundant. This quick lesson saves a lot of pain later. Now what if each x is 2? Or 5? Easy. X can be anything.

By now, it's getting to the "no big deal" stage. Take a look at the problems below; he did them one by one...and approached them with supreme confidence that he could do it. In fact, it was basically a game of solving the puzzles I made.

We certainly didn't start here; but hour after hour--little by little--each concept compounds and we are now at the point where I just write the symbols and he does the rest. Note: I am teaching him much more than factoring. He is still learning addition facts and multiplication facts AT THE SAME TIME he is learning to factor polynomials. He took more than half an hour to do these four problems, and he had to do a lot of math to solve them.
Expect lots of trial and error; he learned several ways that didn't work for these problems but gained information that will come in handy later.
Take the third expression for example:
x2 + 9x + 20.
He discovered several ways to break up 9, before he got to 4 and 5.
4 + 5 = 9 and 4 x 5 = 20,
we also discussed the economy counting disguised as "fewest pieces possible" so we used four 5's instead of five 4's which is still a little amazing to a 7 year old. He figured out right away that two 10's wouldn't work.


...than the fact that he had factored x2 + 11x + 28! I know 17 year olds who run away in fear when confronted with these kinds of problems, and college graduates who can't remember how to do this...but they paid for the credits anyway.
He also drew a picture of each problem. Drawing is crucial for understanding why we use symbols. It brings the point home that the symbols are faster and that the mathematics expresses reality numerically. He won't soon forget because he knows what the symbols mean and what they look like. The hardest part was counting out 28...what to use? 9's? 8's?...ah hah! 7's.
In the process he figured out some multiplication facts for 8's and 9's...on his way to discovering he needed four 7's...he also made comments like, "this number isn't square...if we had 12x we could make a square number..."
Here is a Polynomial PDF to practice all 45 addends and many multiplication facts too.
Most importantly, we had fun the whole time. Since he stayed on task the whole time and since we did a lot of math very quickly, he got some free time to draw. He was much more impressed with his ability to make 8's and 2's than his ability to factor the trinomial! And the pirate ship came out pretty cool too.


Here is a post with lots of video showing ALGEBRA with toddlers.
Tweet
Saturday, August 22, 2009
Experience the Division.

 Here we see a very simple problem of 14/2. We put the blocks on the white board and counted the sides...they can see and feel/touch that there really are 2 sevens in 14.
Here we see a very simple problem of 14/2. We put the blocks on the white board and counted the sides...they can see and feel/touch that there really are 2 sevens in 14.What we are doing is counting how many 7's are contained in 14: there are 2 of them...we counted to 14 one at a time, two at a time and lastly seven at a time. I am quite pleased that the 4 year old can count by 2's and 3's...
A few days ago in the store I gave him a simple real life problem.
If it costs two dollars a week to put a business card on the bulletin board at the health food store, how much will it cost for 4 weeks.
First response, "I dunno that's hard!"
"Stop!" I said. "Let's think about it. Hold up 4 fingers. How many?"
Laugh. "Four...!"
"Okay now each one of your fingers has a two on it..."
He nods vigorously. "Two, four, six...eight! It's eight!"
"Excellent!"
"How old is that kid?"
"I'm four!!"
He didn't have the blocks he had had his imagination. People alwaysraise objections like what happens "when they don't have the blocks?"...or "they can't take the blocks to school..." Yes they most certainly can...in their minds.
 People are thinking he's a little genius. Now, if everybody thinks he's smart, and they treat him like he's smart what are the chances that he will live up to their expectations? If he believes he's smart and can do math now, it will take a lot to shake that belief later.
People are thinking he's a little genius. Now, if everybody thinks he's smart, and they treat him like he's smart what are the chances that he will live up to their expectations? If he believes he's smart and can do math now, it will take a lot to shake that belief later.The younger child simply emulates his brother. It will be even easier for him. He's counting right along with us. How great is his understanding now? Probably not 100%, but a year from now his understanding will be much greater than the average child because he has been exposed to and is comfortable in a math rich environment. A basic tenet: put the child in a language rich environment and they learn that language.
Hawaiian immersion schools prove that. The children learn Hawaiian because they are in a environment that is language rich--all they hear is Hawaiian, they see Hawaiian words, they see the words related the world around them. Math is "just another" language ...

Here we see 9. 3x3, 9/3...
How many three's in 9?
9 can be square...and math being the thinking game it is...fuel of carrots and peanut butter keeps the brain running healthily.
Wednesday, August 19, 2009
90 Min Flies By...

I know I'm doing it right when there isn't any time for me...
Last few sessions I have had, have flown by in an instant. Have a little seven year old doing problems like 12 x 13, 13 x 15 etc...and having fun doing it...because bigger is funner.
Had a couple young girls doing math and having some fun...we did algebra...than backed up to addends...how is it possible we have created a system where a 14 year old girl doesn't know for sure what 6 needs to be ten? It boggles my mind, and the worst part is the system will start blaming HER.
In the picture above we were doing a compound teaching lesson where I showed her 15 x 13 and (x+5)(x+3)...just introducing them to the patterns and later when we evaluate these expressions for various values of x it will be easy. Then we went from multiplication to division it took a second for them to realize we were doing the same problems...

In my defense I wrote those upside down. After this we backed up and built addends for 10 and 9...they had a little race and I could see they have some sisterly competitiveness...which I can gently exploit to get more done in less time. I started the lesson with the 5 concepts then a quick lesson on rounding for their little sister who was not present, they assured me she had it wired. Then we moved onto a quick lesson on where these symbols (greater than and less than) come from
< >
No more confusion or memory devices. They know what the symbols mean. One more time and it should be "in there" [minds] and easily re-callable.
Then came some two digit by two digit multiplication, mostly as a confidence builder and then the algebra and then addends...and then 90 minutes had gone by. To me it felt like 5 minutes max....so I was shocked when their mother said an hour had gone by and pleaded for more time.
Also if you go here you can see the testimonials are trickling in. There seems to be a common theme: FUN.
Sunday, August 16, 2009
Why just teach one thing at a time?

Upon seeing this picture the youngest boy said, "That's us doing our shapes and counting!!" For him that's exactly what it was. For the older boy we were building rectangles and counting the parts....one big red one, five blue ones, two pink ones which are threes so six units. We do addition multiplication and counting all at once. We also pattern for 13 x 12 = 156 and we learn a little place value (count the big ones first).
I was at a party recently, handed someone my card and he said, "Math?!! My kid's only 3 years old." To which I replied, "are you teaching him English?"
Those nearby laughed and nodded...
"Math the way I teach it is a language. Just check out my website. Take what you like leave the rest."
Before this we did more place value. Then we just named the blocks one through ten and of course the hundred even though they already know it...Then did multiplication.
Started with 12 x 13 = 156 and spent time counting all the parts and counting the sides. Look at all the math:

**added 2 tens and 3 tens to get 5 tens
**counted 3 two times to get six
**saw that six can be a rectangle made with 2 threes or 3 twos...
**counted the big one then the blue ones and the units and talked about what each number in 156 meant.
Counted the sides very carefully to get 12 and 13...from 1 to 12 and from 1 to 13. then we counted fast, one ten and three more or one ten and two more is so much faster than counting 1,2,3,4,5,6....
Again they have to be taught to start at 10 even though they supposedly "know" one side of the red square is ten or the blue bar is ten...
Then we flipped them over and did algebra..and we were done...might have spent 20 minutes tops...on the entire lesson.
This compound teaching where each students takes away something different but where more than one concept is introduced at a time. Again the sub-concious mind logs all this information. It can (and will) be drawn forth later.
Wednesday, August 12, 2009
Confidence is key.

Here we have a 14 year old student, bright, above average intellect, likes to read does well in every subject except math. After a very short set of test questions I find damaged self-esteem and self confidence due to math anxiety brought about by previous failures in math. If you look carefully you can see a little bit of this telegraphed in her posture.
Decimals and fractions brought up instant mental blocks even when the problems were seemingly simple. Student brought an example of her pre-algebra which for the moment she has little difficulty doing. This gives me time to work on basics and drill for skills. Here we are working on addends which will certainly help her solve problems like p + 7 = 15.
We covered the five basic concepts. Did a few examples from her homework worksheet. Did some more advanced story problems that required two steps to solve. We then backed up and built tens and nines and did some subtraction. And then moved on to more basics: addends past ten. (Pictured above.)
The idea was to build confidence and get a feel for the students strengths and weaknesses. Strength: exceptionally quick mind. Weakness. ZERO confidence. This will improve as she grows to trust that I am not going to trick her and that the answers will be completely obvious 99.9% of the time. The other .1 may involve some thinking.
Then we did the 11 times table starting at 11 x 11, on up to 11 x 20 she got the pattern instantly then a few 12's and then flipped over to Algebra where she amazed herself by doing problems like (x+2)(x+3) = x2+5x+6...well almost she didn't really think it was hard because she could see it...then oops an hour and a half went by...
will put in many links later...
Sunday, August 9, 2009
Multiplication by song.
So the morning began with a rousing rendition of intsy weentsy spider, which then morphed into Mortensen Math's four song.."You'll never catch a four out to lunch..." And then we moved to Multiplication Rock...and watched all of them. More importantly we sang some of them...everyday they learn more of the songs...which means by the time they are in kindergarten they will have most of their multiplication tables wired. Now that's a head start.
Eat Sleep Math.
Everyday.
Eat Sleep Math.
Everyday.
Wednesday, August 5, 2009
Dyslexic doesn't matter.

Here are a bunch of block towers built into a city. Each block is counted, or skip counted and we practice our multiplication while we do it. If we are using three's we count 3, 6, 9, etc...as we (or they) build the tower.
Originally the plan for this blog was simple: I'd do math, take a few pics and then describe what I did before I went to bed or first thing in the morning...well it isn't quite working out that way. Fortunately I take good notes. I have done quite a few tutoring sessions since my last post here.
Here is what happened during a few of them.
This is a child aged 5 years. Severely dyslexic. Does not like to write or draw, however even in the last week that is beginning to change. He is very sweet and very bright. He also has slight challenges in speech and hearing. We work on saying the numbers as we go along.
Class starts with him putting away a mess of blocks from the student before him.
"How are you?" I ask.
"So good." He replies. And I knew we were going to have a great day.
First things first, put all the blocks away in the tray and count them as we do it. We count 1 thru 9, then on some blocks where he is unsure we just say the name of the block over and over again as he puts it away. We also count the bigger blocks and then put them away.
All blocks put away. Now we build. First we build 10's then 9, 8, and at last 7. He does ALL the building by himself which is progress because he used to have to have me put one block in and then he would find the block that made ten. For example I would get a 9 and he would complete it with a 1. And together we would say ten!!! with great enthusiasm as denoted by the three exclamation points.
Now we have them all built in the tray...time for some exercises. Use one finger to point to the ten now show me the seven and what goes with it?
"Three!!"
Do this for every combination in the tray.
Now it's time for TWO fingers.
Use one finger on each hand to point to the combinations. Get fancy, cross over with his hands in other words point to the six that's on the right with his left hand and the four that's on the left with his right hand.

Now lets build some robots. First we build the thing and then we replace the parts with it's addends. (i.e. if it has a nine in it we replace it with a two and a seven, or a three and a six etc.) This kid likes robots, other kids build other things like pirate ships or rockets or airplanes. Whatever is most fun. In the picture at right we see on robot that has yet to be new and improved and one that has.
Then we built some skyscrapers, which continues to refine his fine motor skills and then we built some towers with three's and we were done. There was a time when he could barely build any of these things and would get frustrated because he would knock the blocks over before he was finished. I have been working with this child for almost 18 months his progress continues to amaze those around him.
We put the blocks away and an hour was gone.
It didn't matter at all that the kid was dyslexic, he could still count and put numbers together and learn the concepts in this lesson; we never even got out a pen and white board, or paper and pencil. Other times we do and the child can barely make a "1" or a "C" much less a "8" or something complicated like "3". Of late he has gotten good at "0" and "1" and has learned to make "9" (a zero on a stick) a "10" and is beginning to make 8's...he often writes "10" as "01" which gives us a lovely opportunity to talk about making sure the numbers are in the right places and place value.
He knows he has a problem because it seems to depress him when it's time to try some drawing. He heaves a big sigh and says "okay" as glumly as possible. He is improving, however. The blocks make it so when the time comes all he has to do is concentrate on making the symbols; the concepts will already be easy. With this method the symbols don't get in the way. In fact we will see that the symbols do what they are supposed to do which is save time. Symbols are faster than drawing or getting out blocks. Dyslexic or not.
It is overwhelming when doing symbol based math to only see 4 + 5 = 9, for example. The symbols are difficult to copy and draw because they don't see what we see, and then they have to remember how many four is, and then they have to remember how many five is, and then they have to add it together and then they have to make the symbols...and then...it's too much. This method breaks it down for them and they learn what the symbols mean. It's easy because the blocks make it visually obvious. We progress in stages; bite sized pieces that are easy to swallow. By the way he can do algebra. We just didn't do any that day..
It really levels the playing field for these children and builds self esteem because they can do the math they just can't always make the symbols but that will be mastered too--just not as quickly as other children his age. Doesn't mean he's special, or more or less than other students just different. I treat him like any other kid and he appreciates that most of the time. (Of course everybody likes special treatment.)
Monday, August 3, 2009
SIX.
Well today's "lesson" for the wee ones was about 6. How many is six...? We counted six. We listen to "I Got Six" from Multiplication Rock. We measured 6 with 2 threes. We measured six with 2's and found out there 3 of them in there. We counted out six units...we made rectangles with the 2's and the 3's. They lost interest. We quit in favor of reading rainbow on PBS.
Wednesday, July 29, 2009
Math lesson 21July2009. Challenged.
This lesson was taught to a bright and vibrant child with some slight challenges in hearing and speech. 5 years old. Fine motor skills undeveloped. Cannot draw or write (YET). He had class after his brother and I made sure that we did not put the blocks away before the new lesson. Therefore his first task was put away blocks.
He sorts and counts blocks as he puts them away. Counting to nine several times as a warm up...then we built 10, 9 , 8, 7, at the lowest degree of difficulty where I put the blocks in first and he just has to put the block that matches in the tray, for example the nine is in the tray and he has to put a one in there to make 10...etc. See picture below. Then he built them himself with no help. But still at the lowest degree of difficulty where he put in one thu 9 or 1 thru 9 etc ad ten added the matching addend. Building them one at a time is the next degree of difficulty.

I then made him use alternating hands to put the blocks in the tray, he could not pick up a block with the same hand twice in a row, thus engaging more of his brain and making it more FUN. He showed me each hand before he grabbed the block he wanted and I nodded and smiled each time giving him positive reinforcement.
Then we made a house with walls that were 10, 9, 8, and seven and eight high (this is not the exact one but you get the idea, this picture shows one a little more advanced with addends past ten.):
Then we counted on our fingers. Two fingers on this hand three on the other: how many?
He had a little trouble putting up his fingers and copying me, but it was fun ad we did lots of combinations less than 5, obviously. 2 + 2, 3 + 2, 4+5, 4+4 etc.
Example: 5 + 3 = 8
He always counted starting at one, even if he knew one hand was 5 and the other 3. He started 1, 2, 3, 4, 5 and then 6, 7, 8. They have to be taught to start at FIVE and then count 6, 7, 8. this takes patience and practice because they always want to start at one just to be sure. We are counting on our fingers now because one day soon we will NOT be counting on our fingers. Fingers are a crutch we will ween off of shortly. Like training wheels on your bike, we won't need them once we get going and it will actually hamper you once you want to go fast and do tricks. We will know these facts "by heart" and the child will know all 45 addends by heart too. He had fun making up his own problems for me to count, part of the fun was getting his fingers to do what he wanted them to do. We then counted to 20 by fives using all our hands...we did this several times.
Many students come to me with an F in algebra and can't add 7 + 8 without either counting on their fingers (as seripticiously as possible) or stopping to think about it. Multiplying 7 x 8 also gives them pause. Fractions poorly understood...gee how come they don't get algebra? But I digress.
Then just for fun we built 3's and 4's because it's super easy, fun and quit because an hour had gone by. Note we never once used paper and pencil or even a white board.
He sorts and counts blocks as he puts them away. Counting to nine several times as a warm up...then we built 10, 9 , 8, 7, at the lowest degree of difficulty where I put the blocks in first and he just has to put the block that matches in the tray, for example the nine is in the tray and he has to put a one in there to make 10...etc. See picture below. Then he built them himself with no help. But still at the lowest degree of difficulty where he put in one thu 9 or 1 thru 9 etc ad ten added the matching addend. Building them one at a time is the next degree of difficulty.

I then made him use alternating hands to put the blocks in the tray, he could not pick up a block with the same hand twice in a row, thus engaging more of his brain and making it more FUN. He showed me each hand before he grabbed the block he wanted and I nodded and smiled each time giving him positive reinforcement.
Then we made a house with walls that were 10, 9, 8, and seven and eight high (this is not the exact one but you get the idea, this picture shows one a little more advanced with addends past ten.):

Then we counted on our fingers. Two fingers on this hand three on the other: how many?
He had a little trouble putting up his fingers and copying me, but it was fun ad we did lots of combinations less than 5, obviously. 2 + 2, 3 + 2, 4+5, 4+4 etc.
Example: 5 + 3 = 8
He always counted starting at one, even if he knew one hand was 5 and the other 3. He started 1, 2, 3, 4, 5 and then 6, 7, 8. They have to be taught to start at FIVE and then count 6, 7, 8. this takes patience and practice because they always want to start at one just to be sure. We are counting on our fingers now because one day soon we will NOT be counting on our fingers. Fingers are a crutch we will ween off of shortly. Like training wheels on your bike, we won't need them once we get going and it will actually hamper you once you want to go fast and do tricks. We will know these facts "by heart" and the child will know all 45 addends by heart too. He had fun making up his own problems for me to count, part of the fun was getting his fingers to do what he wanted them to do. We then counted to 20 by fives using all our hands...we did this several times.
Many students come to me with an F in algebra and can't add 7 + 8 without either counting on their fingers (as seripticiously as possible) or stopping to think about it. Multiplying 7 x 8 also gives them pause. Fractions poorly understood...gee how come they don't get algebra? But I digress.
Then just for fun we built 3's and 4's because it's super easy, fun and quit because an hour had gone by. Note we never once used paper and pencil or even a white board.
Math lesson 21July2009.
Been busy. Have done hours of tutoring since my last post.
Here is a description of what happened for one of of those hours.
Student 7 years old. Normal to above average intelligence. Slight hyperactivity. Expressed in hyperkenetic activity and extreme talkativeness. He's a regular kid.
First we built tens on down to two's.
Then we talked about subtraction, and with the blocks right there verbally drilled him on facts. 10 take away 4, 8 kids in the room, three leave how many left? Etc. Did 15 different facts and five that were the same. Did not relate subtraction and addition. In other words 10 - 4 = 6 Is exactly the same problem as 6 need what to be ten...when you have the blocks. He had the tray to look at the whole time. So it was easy. Soon he will only have symbols...but first he will be absolutely confortable with blocks and drawing.
Then we built rectangles and labeled the sides and hid the answers underneath. The over the shoulder shot show him labeling 5 eights...


Then we talked about division and multiplication. Here we see symbols only and we have adjusted the symbols to fit the blocks the way we wanted. You don't see that we changed the sevens to show five across 7 down and used 5's because 5 is contained in 35, seven times. We also talked about how to read that expression...4 IS CONTAINED IN 16 four times. He could see that, it was visually obvious.

We then added the arrows for the pattern across and down. and as you can see he got the blocks out to help him. All he had to do was count the down he already knew the one side and how many in the rectangle, I thought he would get out 5's but he got out 4's because he knew the answer. That problem says build 20 with fives. He was in a situation where he could not fail. So he was confident. The important part was he got it right and understood that he had to count the other side. He also understood 4 is contained in 20 five times. Multiplication and division all at once. They are inverse functions after all.
Then we moved on to his favorite topic: ALGEBRA! We moved on to cross teaching or compound teaching concepts. The first "problem" was make as many rectangles as you can with x2+ 7x...
So he built
x2+ 7x + 10
x2+ 7x + 12
x2+ 7x + 6
We talked briefly about the factors (ie the factors of x2+ 7x + 10 are (x + 2)(x + 5) ) but mostly we were concerned with the 10 being made up of 2 fives or 5 twos.

Then we added 3 more x. It was a tiny problem how many more x do we need? We had 7x so we needed 3x to make 10x. Algebra is easy! Now we made as many rectangles as we could with
x2+ 10x
He started out with x2+ 10x + 25
then did x2+ 10x + 21 then x2+ 10x +16 which you see pictured here. He has 2 on top and 8 on the side. (x + 8) across and (x + 2) up.
x2+ 10x + 16 = (x + 8) (x + 2).
We were more focused on the 2 x 8 part. The algebra is a bonus as it were. (!) It was much faster to make it with two 8's than with eight 2's. This is known as the economy of counting. We discussed this concept only briefly. But it's obvious it's faster to count 8, 16, than 2, 4, 6, 8, 10, 12, 14, 16...we did both of course.
Then x2+ 10x + 24 and lastly x2+ 10x + 9. Note how much math is going on here and why I call it compound teaching. He is learning all the addends for 10, the factors of the algebraic expressions and some the factors of the numbers 25, 21, 16, 24 and 9. We are working on all of this all at once and having fun doing it. Nothing scary or hard about it.
The we continued building the addends to 18...
Then the hour was up. 60 minutes flew by.
Here is a description of what happened for one of of those hours.
Student 7 years old. Normal to above average intelligence. Slight hyperactivity. Expressed in hyperkenetic activity and extreme talkativeness. He's a regular kid.
First we built tens on down to two's.
Then we talked about subtraction, and with the blocks right there verbally drilled him on facts. 10 take away 4, 8 kids in the room, three leave how many left? Etc. Did 15 different facts and five that were the same. Did not relate subtraction and addition. In other words 10 - 4 = 6 Is exactly the same problem as 6 need what to be ten...when you have the blocks. He had the tray to look at the whole time. So it was easy. Soon he will only have symbols...but first he will be absolutely confortable with blocks and drawing.
Then we built rectangles and labeled the sides and hid the answers underneath. The over the shoulder shot show him labeling 5 eights...


Then we talked about division and multiplication. Here we see symbols only and we have adjusted the symbols to fit the blocks the way we wanted. You don't see that we changed the sevens to show five across 7 down and used 5's because 5 is contained in 35, seven times. We also talked about how to read that expression...4 IS CONTAINED IN 16 four times. He could see that, it was visually obvious.

We then added the arrows for the pattern across and down. and as you can see he got the blocks out to help him. All he had to do was count the down he already knew the one side and how many in the rectangle, I thought he would get out 5's but he got out 4's because he knew the answer. That problem says build 20 with fives. He was in a situation where he could not fail. So he was confident. The important part was he got it right and understood that he had to count the other side. He also understood 4 is contained in 20 five times. Multiplication and division all at once. They are inverse functions after all.
Then we moved on to his favorite topic: ALGEBRA! We moved on to cross teaching or compound teaching concepts. The first "problem" was make as many rectangles as you can with x2+ 7x...
So he built
x2+ 7x + 10
x2+ 7x + 12
x2+ 7x + 6
We talked briefly about the factors (ie the factors of x2+ 7x + 10 are (x + 2)(x + 5) ) but mostly we were concerned with the 10 being made up of 2 fives or 5 twos.

Then we added 3 more x. It was a tiny problem how many more x do we need? We had 7x so we needed 3x to make 10x. Algebra is easy! Now we made as many rectangles as we could with
x2+ 10x
He started out with x2+ 10x + 25
then did x2+ 10x + 21 then x2+ 10x +16 which you see pictured here. He has 2 on top and 8 on the side. (x + 8) across and (x + 2) up.
x2+ 10x + 16 = (x + 8) (x + 2).
We were more focused on the 2 x 8 part. The algebra is a bonus as it were. (!) It was much faster to make it with two 8's than with eight 2's. This is known as the economy of counting. We discussed this concept only briefly. But it's obvious it's faster to count 8, 16, than 2, 4, 6, 8, 10, 12, 14, 16...we did both of course.
Then x2+ 10x + 24 and lastly x2+ 10x + 9. Note how much math is going on here and why I call it compound teaching. He is learning all the addends for 10, the factors of the algebraic expressions and some the factors of the numbers 25, 21, 16, 24 and 9. We are working on all of this all at once and having fun doing it. Nothing scary or hard about it.
The we continued building the addends to 18...
Then the hour was up. 60 minutes flew by.